Adjustable Stoles: Resizing Rectangle Shawls

Welcome to Day 4 of the Adjustable Shawls series! Today, we’re talking about adjustable stoles and resizing rectangle shawls.

The Basics of Rectangle Shawls (Stoles)

There is a whole bunch of information about stoles available in my Shawl Design For Everybody series here on knitting.today. If you don’t know anything about rectangular shawls and how they are constructed yet, feel free to browse the series and learn about

• Rectangular Shawls (Stoles)

in the shawl design online series before proceeding.

Resizing Rectangle Shawls

Stoles are adjustable by default when worked hem to hem or from center out. Make sure you use your kitchen scale as described in the post Your Most Important Tool when working from center outwards or with patterned stoles.

For alignment of pattern modules in square shawls the same principles as outlined in the post on the math of adjustable shawls – What Makes Shawls Adjustable? – apply here, too.

Pattern Modules in Stoles

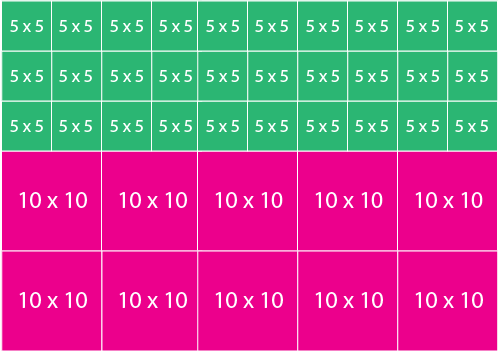
An example for a stole module setup is shown below: the modules used are a 5 by 5 and a 10 by 10 stitches pattern repeat.

No matter how many rows of 10 x 10 modules are worked, the stitch count is always 50 stitches (five 10 x 10 modules). As 5 x 5 modules can be fitted into a 50 stitch row without remainders, this shawl is adjustable without any problem.

If you want to combine 10 x 10 with 7 x 7 panels, things look a bit differently.

All combinations of 10 x 10 panels give stitch counts divisible by 10: working a number N of 10 x 10 panels results in a stitch count of N*10.

All combinations of 7 x 7 panels give stitch counts divisible by 7: working a number M of 7 x 7 panels results in a stitch count of M*7.

The trick is to find specific numbers M and N for which N*10 = M*7 is true.

The solution is relatively easy here: N*10 = 10*N which has to be equal to M*7 to make it work:

10*N = M*7

Can you spot the solution? If N = 7 and M = 10, the equation is true:

10 * 7 = 10 * 7 = 70.

We need a stitch count of 70 to make it work! So if we choose to work 7 repeats of 10 x 10 panels and 10 repeats of 7 x 7 panels, it works out.

It also works out for each multiple: 2 * (10 * 7) = 140 stitches, 14 repeats of 10 x 10 panels, works too. And also 3 * (10 * 7) = 210, 4 * (10 * 7) = 210, and so on. If you still have no clue what I’m talking about, get out your undergrad math books and look up the chapter on the least common multiple. (Do you still wonder why the heck you had to learn this awkward math things in school? Now here you got your answer! You can use them to make your knitting life easier!)

The easiest formula for a working combination of A x A with B x B stitch panels is to calculate your needed stitch count by using

C * (A * B)

where C is any whole number greater than zero (1, 2, 3, …) and A and B are your panel stitch counts. (Cave: this works for symmetrical panels only where the stitch count equals the row count in one panel! It does NOT work for panels A x B where A is not equal to B.)

Summary

Stoles using only one stitch pattern (for instance, only use garter stitch or only one pattern repeat) are adjustable by default. Resizing rectangle shawls is easy in this case.

To turn stoles with different stitch patterns into adjustable stoles, you have to make sure the stitch count before and after a pattern transition (changing the pattern within the stole) does not change.

Any questions so far? If yes, feel free to drop me a comment!

Save

Save

Save